Humans have a predisposition for numbers between 1 and 10. This is surely not a coincidence, given that most of us learn to count on our fingers.

Numbers larger than 10 tend to get lumped together as “really big numbers.” Numbers less than 1 tend to get lumped together as “really small numbers.”

Let’s look at a few examples, shall we? Here are some approximate distances in kilometers (km):

60: Washington, D.C to Baltimore

3700: Washington, D.C. to Los Angeles, California

384,000: Earth to moon

150,000,000: Earth to Sun

40,000,000,000,000: Our Sun to Proxima Centauri, our nearest neighboring star

920,000,000,000,000,000,000,000: Diameter of observable universe

One can maintain a sense of scale through a few hundred or perhaps a few thousand kilometers. However, beyond a certain number of zeroes, it just looks like a lot of zeroes and all the numbers just look “really big” and it gets difficult to tell how much bigger one “really big” number is than another.

Now let’s take some examples of small numbers. Here are some approximate dimensions in meters (m):

1.7: Typical Height of a human

0.005: Typical length of a red ant

0.0005: Typical diameter of a human egg cell

0.00001: Typical diameter of a human red blood cell

0.000000002: Diameter of DNA helix

0.00000000005: Radius of hydrogen atom

0.000000000000001: Diameter of proton or neutron

One can maintain a sense of scale through perhaps 3 or 4 places to the right of the decimal point. However, beyond that, the numbers all just look “really small” and it gets difficult to tell how much smaller one “really small” number is than another.

Enter Scientific Notation and Powers of Ten.

In Scientific Notation, any number, no matter how large or small, can be expressed as a number with one digit to the left of the decimal point (i.e. a number between 1 and darned close to 10) multiplied by a power of 10. (If you have not read the Exponents page, this would be a good time to do so. )

When the exponent of 10 is a positive integer, the exponent defines the number of zeroes that come after the 1:

10¹ = 10

10² = 100

10³ = 1000

10⁶ = 1,000,000

10⁹ = 1,000,000,000

etc.

When the exponent of 10 is a negative integer, the exponent defines the number of places to move the decimal point to the left of the 1:

10^-1 = 0.1

10^-2 = 0.01

10^-3 = 0.001

10^-6 = 0.000001

etc.

And let’s not forget:

10⁰ = 1

In Scientific Notation, the exponent of 10 defines the direction and the number of places to move the decimal point (to the right for a positive exponent, to the left for a negative exponent).

The examples of large numbers above convert to Scientific Notation as follows (all distances in km):

60 = 6 x 10¹: Washington, D.C to Baltimore

3700 = 3.7 x 10³: Washington, D.C. to Los Angeles, California

384,000 = 3.84 x 10⁵: Earth to moon

150,000,000 = 1.5 x 10⁸: Earth to Sun

40,000,000,000,000 = 4 x 10¹³: Our Sun to Proxima Centauri, our nearest neighboring star

920,000,000,000,000,000,000,000 = 9.2 x 10²³: Diameter of observable universe

Now, instead of looking at a bunch of zeroes, we can make quantitative comparisons more readily. The distance from the Earth to the Sun is about 3 powers of 10 (factor of 1000) greater than the distance from the Earth to the moon (10⁸ vs. 10⁵).

A commonly used synonym for “power of 10” is “order of magntitude.” It is equivalent to say that the distance from the Earth to the Sun is 3 orders of magnitude greater than the distance between the Earth and the moon.

Similarly, the distance from our Sun to Proxima Centauri is about 5 orders of magnitude (i.e. 5 powers of ten = 100,000) greater than the distance from the Earth to the Sun (10¹³ vs. 10⁸).

The examples of small numbers above convert to Scientific Notation as follows (all distances in meters):

1.7 = 1.7 x 10⁰: Typical height of a human

0.005 = 5 x 10^-3: Typical length of a red ant

0.0005 = 5 x 10^-4: Typical diameter of a human egg cell

0.00001 = 1 x 10^-5: Typical diameter of a human red blood cell

0.000000002 = 2 x 10^-9: Diameter of DNA helix

0.00000000005 = 5 x 10^-11: Radius of hydrogen atom

0.000000000000001 = 1 x 10^-15: Diameter of proton or neutron

Again, relative comparisons may be made much more efficiently by comparing exponents instead of by counting decimal places. For example, a red blood cell is about 5 orders of magnitude smaller than a typical human; the diameter of a DNA helix is about 4 orders of magnitude smaller than a red blood cell; a proton is about 4 orders of magnitude smaller than a hydrogen atom.

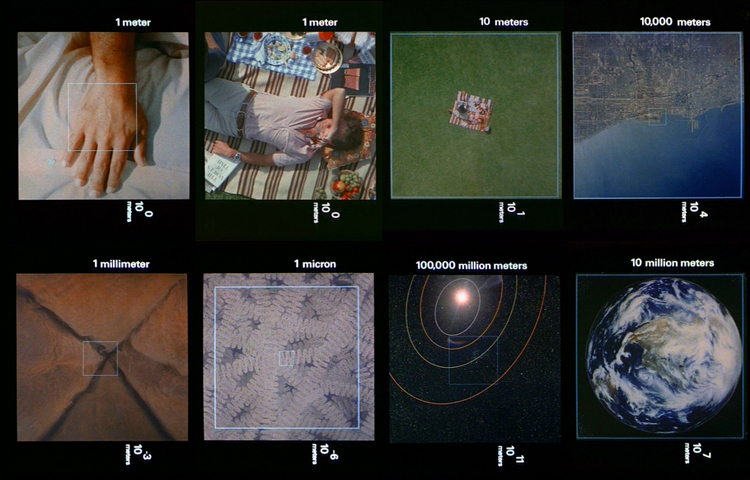
The 1977 film “Powers of Ten,” by Charles and Ray Eames for IBM, provides a wonderful illustration of this concept to show the relative sizes of objects in the universe, from the very large to the very small. Despite being made long before computer graphics were readily available, the film is very well done, it looks good, it has stood the test of time, and it is well worth watching if you have never seen it.

The film is 9 minutes long and may be viewed below (be forewarned – Pluto was still considered a planet in 1977):